Multiple Integrals
Double Integrals Over Rectangles
For a rectangular region [latex]S[/latex] defined by [latex]x[/latex] in [latex][a,b][/latex] and [latex]y[/latex] in [latex][c,d][/latex], the double integral of a function [latex]f(x,y)[/latex] in this region is given as [latex]\int_c^d(\int_a^b f(x,y) dx) dy[/latex].Learning Objectives
Use double integrals to find the volume of rectangular regions in the xy-planeKey Takeaways
Key Points
- The multiple integral is a type of definite integral extended to functions of more than one real variable —for example, [latex]f(x, y)[/latex] or [latex]f(x, y, z)[/latex]. Integrals of a function of two variables over a region in [latex]R^2[/latex] are called double integrals.
- The double integral of a positive function of two variables represents the volume of the region between the surface defined by the function (on the three dimensional Cartesian plane where [latex]z = f(x, y))[/latex] and the plane which contains its domain.
- If there are more variables than 3, a multiple integral will yield hypervolumes of multi-dimensional functions.
Key Terms
- Fubini's theorem: a result which gives conditions under which it is possible to compute a double integral using iterated integrals
- hypervolume: a volume in more than three dimensions
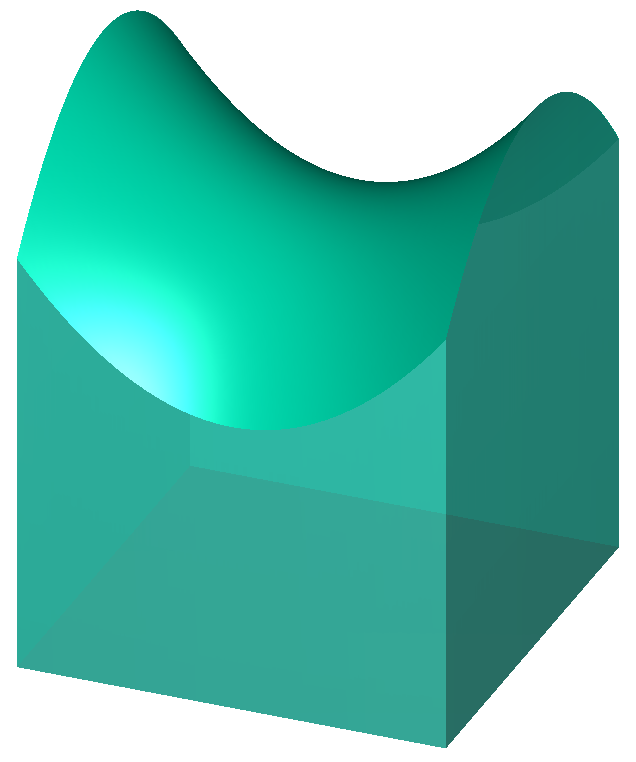
Volume to be Integrated: Double integral as volume under a surface [latex]z = x^2 − y^2[/latex]. The rectangular region at the bottom of the body is the domain of integration, while the surface is the graph of the two-variable function to be integrated.
Double Integrals Over Rectangles
Double integrals over rectangular regions are straightforward to compute in many cases. For a rectangular region [latex]S[/latex] defined by [latex]x[/latex] in [latex][a,b][/latex] and [latex]y[/latex] in [latex][c,d][/latex], the double integral of a function [latex]f(x,y)[/latex] in this region is given as: [latex]\begin{align}\int\!\!\!\int_S f(x,y) dxdy &= \int_a^b\left(\int_c^d f(x,y) dy\right) dx \\ &= \int_c^d\left(\int_a^b f(x,y) dx\right) dy\end{align}[/latex]. Here, we exchanged the order of the integration, assuming that [latex]f(x,y)[/latex] satisfies the conditions to apply Fubini's theorem.Example
Let us assume that we wish to integrate a multivariable function [latex]f[/latex] over a region [latex]A[/latex]: [latex-display]A = \left \{ (x,y) \in \mathbf{R}^2: 11 \le x \le 14 \; \ 7 \le y \le 10 \right \}[/latex-display] [latex-display]f(x,y) = x^2 + 4y[/latex-display] Formulating the double integral, we first evaluate the inner integral with respect to [latex]x[/latex]: [latex-display]\begin{align} \int_{11}^{14} (x^2 + 4y) \ dx & = \left (\frac{1}{3}x^3 + 4yx \right)\Big |_{x=11}^{x=14} \\ & = \frac{1}{3}(14)^3 + 4y(14) - \frac{1}{3}(11)^3 - 4y(11) \\ &= 471 + 12y \end{align}[/latex-display] We then integrate the result with respect to [latex]y[/latex]: [latex-display]\begin{align} \int_7^{10} (471 + 12y) \ dy & = (471y + 6y^2)\big |_{y=7}^{y=10} \\ & = 471(10)+ 6(10)^2 - 471(7) - 6(7)^2 \\ &= 1719 \end{align}[/latex-display] We could have computed the double integral starting from the integration over [latex]y[/latex]. Confirm yourself that the result is the same.Iterated Integrals
An iterated integral is the result of applying integrals to a function of more than one variable.Learning Objectives
Use iterated integrals to integrate a function with more than one variableKey Takeaways
Key Points
- The function [latex]f(x,y)[/latex], if [latex]y[/latex] is considered a given parameter, can be integrated with respect to [latex]x[/latex] as follows: [latex]\int f(x,y)dx[/latex].
- The result is a function of [latex]y[/latex] and therefore its integral can be considered again. If this is done, the result is the iterated integral [latex]\int\left(\int f(x,y)\,dx\right)\,dy[/latex].
- It is key to note that this is different, in principle, to the multiple integral [latex]\iint f(x,y)\,dx\,dy[/latex].
Key Terms
- Fubini's theorem: a result which gives conditions under which it is possible to compute a double integral using iterated integrals

Use of an iterated integral: An iterated integral can be used to find the volume of the object in the figure.
Example
For the iterated integral [latex]\int\left(\int (x+y) \, dx\right) \, dy[/latex], the integral [latex]\int (x+y) \, dx = \frac{x^2}{2} + yx[/latex] is computed first. The result is then used to compute the integral with respect to [latex]y[/latex]: [latex-display]\displaystyle{\int \left(\frac{x^2}{2} + yx \right) \, dy = \frac{yx^2}{2} + \frac{xy^2}{2}}[/latex-display] It should be noted, however, that this example omits the constants of integration. After the first integration with respect to [latex]x[/latex], we would rigorously need to introduce a "constant" function of [latex]y[/latex]. That is, If we were to differentiate this function with respect to [latex]x[/latex], any terms containing only [latex]y[/latex] would vanish, leaving the original integral. Similarly for the second integral, we would introduce a "constant" function of [latex]x[/latex], because we have integrated with respect to [latex]y[/latex]. In this way, indefinite integration does not make much sense for functions of several variables. While the antiderivatives of single variable functions differ at most by a constant, the antiderivatives of multivariable functions differ by unknown single-variable terms, which could have a drastic effect on the behavior of the function.Double Integrals Over General Regions
Double integrals can be evaluated over the integral domain of any general shape.Learning Objectives
Use double integrals to integrate over general regionsKey Takeaways
Key Points
- If the domain [latex]D[/latex] is normal with respect to the [latex]x[/latex]- axis, and [latex]f:D \to R[/latex] is a continuous function, then [latex]\alpha(x)[/latex] and [latex]\beta(x)[/latex] (defined on the interval [latex][a, b][/latex]) are the two functions that determine [latex]D[/latex]: [latex]\iint_D f(x,y)\ dx\, dy = \int_a^b dx \int_{ \alpha (x)}^{ \beta (x)} f(x,y)\, dy[/latex]
- Applying this general method, the projection of [latex]D[/latex] onto either the [latex]x[/latex]-axis or the [latex]y[/latex]-axis should be bounded by the two values, [latex]a[/latex] and [latex]b[/latex].
- For a domain [latex]D = \{ (x,y) \in \mathbf{R}^2 \: \ x \ge 0, y \le 1, y \ge x^2 \}[/latex], we can write the integral over [latex]D[/latex] as[latex]\iint_D (x+y) \, dx \, dy = \int_0^1 dx \int_{x^2}^1 (x+y) \, dy[/latex].
Key Terms
- domain: the set of all possible mathematical entities (points) where a given function is defined
- the projection of [latex]D[/latex] onto either the [latex]x[/latex]-axis or the [latex]y[/latex]-axis is bounded by the two values, [latex]a[/latex] and [latex]b[/latex].
- any line perpendicular to this axis that passes between these two values intersects the domain in an interval whose endpoints are given by the graphs of two functions, [latex]\alpha[/latex] and [latex]\beta[/latex].
Example
Consider the following region: [latex-display]D = \{ (x,y) \in \mathbf{R}^2 \: \ x \ge 0, y \le 1, y \ge x^2 \}[/latex-display] Calculate [latex]\iint_D (x+y) \, dx \, dy[/latex]. This domain is normal with respect to both the [latex]x[/latex]- and [latex]y[/latex]-axes. To apply the formulae, you must first find the functions that determine [latex]D[/latex] and the intervals over which these are defined. In this case the two functions are [latex]\alpha (x) = x^2[/latex] and [latex]\beta (x) = 1[/latex], while the interval is given by the intersections of the functions with [latex]x=0[/latex], so the interval is [latex][a,b] = [0,1][/latex] (normality has been chosen with respect to the [latex]x[/latex]-axis for a better visual understanding).
Double Integral: Double integral over the normal region [latex]D[/latex] shown in the example.
Double Integrals in Polar Coordinates
When domain has a cylindrical symmetry and the function has several specific characteristics, apply the transformation to polar coordinates.Learning Objectives
Solve double integrals in polar coordinatesKey Takeaways
Key Points
- The fundamental relation to make the transformation is the following: [latex]f(x,y) \rightarrow f(\rho \cos \varphi,\rho \sin \varphi )[/latex].
- To switch the integral from Cartesian to polar coordinates, the [latex]dx \,\, dy[/latex] differentials in this transformation become [latex]\rho \,\, d\rho \,\,d\varphi[/latex].
- Once the function is transformed and the domain evaluated, it is possible to define the formula for the change of variables in polar coordinates: [latex]\iint_D f(x,y) \ dx\,\, dy = \iint_T f(\rho \cos \varphi, \rho \sin \varphi) \rho \,\, d \rho\,\, d \varphi[/latex].
Key Terms
- Cartesian: of or pertaining to co-ordinates based on mutually orthogonal axes
- Jacobian determinant: the determinant of the Jacobian matrix

Transformation to Polar Coordinates: This figure illustrates graphically a transformation from cartesian to polar coordinates
Change of variable
The polar coordinates [latex]r[/latex] and [latex]\varphi[/latex] can be converted to the Cartesian coordinates [latex]x[/latex] and [latex]y[/latex] by using the trigonometric functions sine and cosine: [latex-display]x = r \cos \varphi \, \\ y = r \sin \varphi \,[/latex-display] The Cartesian coordinates [latex]x[/latex] and [latex]y[/latex] can be converted to polar coordinates [latex]r[/latex] and [latex]\varphi[/latex] with [latex]r \geq 0[/latex] and [latex]\varphi[/latex] in the interval [latex](−\pi, \pi][/latex]: [latex-display]r = \sqrt{x^2 + y^2} \\ \varphi = \tan ^{-1} (\frac yx)[/latex-display] The fundamental relation to make the transformation is as follows: [latex-display]f(x,y) \rightarrow f(\rho \cos \phi,\rho \sin \phi )[/latex-display]Examples
- Given the function [latex]f(x,y) = x + y[/latex] and applying the transformation, one obtains [latex]f(\rho, \phi) = \rho \cos \phi + \rho \sin \phi = \rho(\cos \phi + \sin \phi )[/latex].
- Given the function [latex]f(x,y) = x^2 + y^2[/latex], one can obtain [latex]f(\rho, \phi) = \rho^2 (\cos^2 \phi + \sin^2 \phi) = \rho^2[/latex] using the Pythagorean trigonometric identity, which is very useful to simplify this operation. Particularly in this case, you can see that the representation of the function f became simpler in polar coordinates. This is the case because the function has a cylindrical symmetry. In general, the best practice is to use the coordinates that match the built-in symmetry of the function.
Integrals in Polar Coordinates
The Jacobian determinant of that transformation is the following: [latex-display]\displaystyle{\frac{\partial (x,y)}{\partial (\rho, \phi)}} = \begin{vmatrix} \cos \phi & - \rho \sin \phi \\ \sin \phi & \rho \cos \phi \end{vmatrix} = \rho[/latex-display] which has been obtained by inserting the partial derivatives of [latex]x = \rho \cos(\varphi)[/latex], [latex]y = \rho \sin(\varphi)[/latex] in the first column with respect to [latex]\rho[/latex] and in the second column with respect to [latex]\varphi[/latex], so the [latex]dx \, dy[/latex] differentials in this transformation become [latex]\rho \,d \rho \,d\varphi[/latex]. Once the function is transformed and the domain evaluated, it is possible to define the formula for the change of variables in polar coordinates: [latex-display]\iint_D f(x,y)dx \, dy = \iint_T f(\rho \cos \varphi, \rho \sin \varphi)\rho[/latex-display]Example
Integrate the function [latex]f(x,y) = x[/latex] over the domain: [latex-display]D = \{ x^2 + y^2 \le 9, \ x^2 + y^2 \ge 4, \ y \ge 0 \}[/latex-display] From [latex]f(x,y) = x \longrightarrow f(\rho,\phi) = \rho \cos \phi[/latex], [latex-display]\begin{align} \iint_D x \, dx\, dy &= \iint_T \rho \cos \phi \rho \, d\rho\, d\phi \\ &= \int_0^\pi \int_2^3 \rho^2 \cos \phi \, d \rho \, d \phi \\ &= \int_0^\pi \cos \phi \ d \phi \left[ \frac{\rho^3}{3} \right]_2^3 \\ &= \left[ \sin \phi \right]_0^\pi \ \left(9 - \frac{8}{3} \right) = 0 \end{align}[/latex-display]Triple Integrals in Cylindrical Coordinates
When the function to be integrated has a cylindrical symmetry, it is sensible to integrate using cylindrical coordinates.Learning Objectives
Evaluate triple integrals in cylindrical coordinatesKey Takeaways
Key Points
- Switching from Cartesian to cylindrical coordinates, the transformation of the function is made by the following relation [latex]f(x,y,z) \rightarrow f(\rho \cos \varphi, \rho \sin \varphi, z)[/latex].
- In switching to cylindrical coordinates, the [latex]dx\, dy\, dz[/latex] differentials in the integral become [latex]\rho \, d\rho \,d\varphi \,dz[/latex].
- Therefore, an integral evaluated in Cartesian coordinates can be switched to an integral in cylindrical coordinates as[latex]\iiint_D f(x,y,z) \, dx\, dy\, dz = \iiint_T f(\rho \cos \varphi, \rho \sin \varphi, z)\rho \, d\rho \,d\varphi \,dz[/latex].
Key Terms
- differential: an infinitesimal change in a variable, or the result of differentiation
- cylindrical coordinate: a three-dimensional coordinate system that specifies point positions by the distance from a chosen reference axis, the direction from the axis relative to a chosen reference direction, and the distance from a chosen reference plane perpendicular to the axis

Cylindrical Coordinates: Cylindrical coordinates are often used for integrations on domains with a circular base.
Example 1
The region is: [latex-display]D = \{ x^2 + y^2 \le 9, \ x^2 + y^2 \ge 4, \ 0 \le z \le 5 \}[/latex-display] If the transformation is applied, this region is obtained: [latex-display]T = \{ 2 \le \rho \le 3, \ 0 \le \varphi \le 2\pi, \ 0 \le z \le 5 \}[/latex-display] because the z component is unvaried during the transformation, the [latex]dx\, dy\, dz[/latex] differentials vary as in the passage in polar coordinates: therefore, they become: [latex]\rho \, d\rho \,d\varphi \,dz[/latex]. Finally, it is possible to apply the final formula to cylindrical coordinates: [latex-display]\displaystyle{\iiint Df(x,y,z)dx\,dy\,dz=\iiint Tf(\rho\cos\varphi,\rho\sin\varphi,z)\rho\, d\rho \,d\varphi \,dz}[/latex-display] This method is convenient in case of cylindrical or conical domains or in regions where it is easy to individuate the [latex]z[/latex] interval and even transform the circular base and the function.Example 2
The function [latex]f(x,y,z) = x^2 + y^2 + z[/latex] is and as integration domain this cylinder: [latex-display]D = \{ x^2 + y^2 \le 9, \ -5 \le z \le 5 \}[/latex-display] The transformation of [latex]D[/latex] in cylindrical coordinates is the following: [latex-display]T = \{ 0 \le \rho \le 3, \ 0 \le \phi \le 2 \pi, \ -5 \le z \le 5 \}[/latex-display] while the function becomes: [latex-display]f(\rho \cos \varphi, \rho \sin \varphi, z) = \rho^2 + z[/latex-display] Therefore, the integral becomes: [latex]\begin{align}\displaystyle{\iiint_D (x^2 + y^2 +z) \, dx\, dy\, dz} &\displaystyle{= \iiint_T ( \rho^2 + z) \rho \, d\rho\, d\phi\, dz} \\ &= \int_{-5}^5 dz \int_0^{2 \pi} d\phi \int_0^3 ( \rho^3 + \rho z )\, d\rho\\ &= 2 \pi \int_{-5}^5 \left[ \frac{\rho^4}{4} + \frac{\rho^2 z}{2} \right]_0^3 \, dz\\ &= 2 \pi \int_{-5}^5 \left( \frac{81}{4} + \frac{9}{2} z\right)\, dz\\ &= 405 \pi \end{align}[/latex]Triple Integrals in Spherical Coordinates
When the function to be integrated has a spherical symmetry, change the variables into spherical coordinates and then perform integration.Learning Objectives
Evaluate triple integrals in spherical coordinatesKey Takeaways
Key Points
- Switching from Cartesian to spherical coordinates, the function is transformed by this relation: [latex]f(x,y,z) \longrightarrow f(\rho \cos \theta \sin \varphi, \rho \sin \theta \sin \varphi, \rho \cos \varphi)[/latex].
- For the transformation, the [latex]dx\, dy\, dz[/latex] differentials in the integral are transformed to [latex]\rho^2 \sin \varphi \, d\rho \,d\varphi \,dz[/latex].
- Therefore, an integral evaluated in Cartesian coordinates can be switched to an integral in spherical coordinates as [latex]\iiint_D f(x,y,z) \, dx\, dy\, dz = \iiint_T f(\rho \sin \varphi \cos \theta, \rho \sin \varphi \sin \theta, \rho \cos \varphi) \rho^2 \sin \varphi \, d\rho\, d\theta\, d\varphi.[/latex]
Key Terms
- spherical coordinate: a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its polar angle measured from a fixed zenith direction, and the azimuth angle of its orthogonal projection on a reference plane that passes through the origin and is orthogonal to the zenith
- Jacobian determinant: the determinant of the Jacobian matrix

Spherical Coordinates: Spherical coordinates are useful when domains in [latex]R^3[/latex] have spherical symmetry.
Integrals in Spherical Coordinates
The Jacobian determinant of this transformation is the following: [latex-display]\displaystyle{\frac{\partial (x,y,z)}{\partial (\rho, \theta, \varphi)}} = \begin{vmatrix} \cos \theta \sin \varphi & - \rho \sin \theta \sin \varphi & \rho \cos \theta \cos \varphi \\ \sin \theta \sin \varphi & \rho \cos \theta \sin \varphi & \rho \sin \theta \cos \varphi \\ \cos \varphi & 0 & - \rho \sin \varphi \end{vmatrix} = \rho^2 \sin \varphi[/latex-display] The [latex]dx\, dy\, dz[/latex] differentials therefore are transformed to [latex]\rho^2 \sin \varphi \, d\rho \,d\varphi \,dz[/latex]. Finally, you obtain the final integration formula: It's better to use this method in case of spherical domains and in case of functions that can be easily simplified, by the first fundamental relation of trigonometry, extended in [latex]R^3[/latex]; in other cases it can be better to use cylindrical coordinates.Example
Integrate [latex]f(x,y,z) = x^2 + y^2 + z^2[/latex] over the domain [latex]D = x^2 + y^2 + z^2 \le 16[/latex]. In spherical coordinates: [latex-display]f(\rho \sin \varphi \cos \theta, \rho \sin \varphi \sin \theta, \rho \cos \varphi) = \rho^2[/latex-display] while the intervals of the transformed region [latex]T[/latex] from [latex]D[/latex]: [latex-display]0 \leq \rho \leq 4, 0 \leq \varphi \leq \pi, 0 \leq \theta \leq 2\pi[/latex-display] Therefore: [latex-display]\begin{align} \iiint_D (x^2 + y^2 +z^2) \, dx\, dy\, dz &= \iiint_T \rho^2 \ \rho^2 \sin \theta \, d\rho\, d\theta\, d\phi, \\ &= \int_0^{\pi} \sin \phi \,d\phi \int_0^4 \rho^4 d \rho \int_0^{2 \pi} d\theta \\ &= 2 \pi \int_0^{\pi} \sin \phi \left[ \frac{\rho^5}{5} \right]_0^4 \, d \phi \\ &= 2 \pi \left[ \frac{\rho^5}{5} \right]_0^4 \left[- \cos \phi \right]_0^{\pi}= \frac{4096 \pi}{5} \end{align}[/latex-display]Triple Integrals
For [latex]T \subseteq R^3[/latex], the triple integral over [latex]T[/latex] is written as [latex]\iiint_T f(x,y,z)\, dx\, dy\, dz[/latex].Learning Objectives
Use triple integrals to integrate over three-dimensional regionsKey Takeaways
Key Points
- By convention, the triple integral has three integral signs (and a double integral has two integral signs); this is a notational convention which is convenient when computing a multiple integral as an iterated integral.
- If [latex]T[/latex] is a domain that is normal with respect to the [latex]xy[/latex]-plane and determined by the functions [latex]\alpha (x,y)[/latex] and [latex]\beta(x,y)[/latex], then [latex]\iiint_T f(x,y,z) \ dx\, dy\, dz = \iint_D \int_{\alpha (x,y)}^{\beta (x,y)} f(x,y,z) \, dz dx dy[/latex].
- To integrate a function with spherical symmetry such as [latex]f(x,y,z) = x^2 + y^2 + z^2[/latex], consider changing integration variable to spherical coordinates.
Key Terms
- spherical coordinate: a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its polar angle measured from a fixed zenith direction, and the azimuth angle of its orthogonal projection on a reference plane that passes through the origin and is orthogonal to the zenith

Graphical Representation of a Triple Integral: Example of domain in [latex]R^3[/latex] that is normal with respect to the [latex]xy[/latex]-plane.
Example 1
The volume of the parallelepiped of sides 4 by 6 by 5 may be obtained in two ways:- By calculating the double integral of the function [latex]f(x, y) = 5[/latex] over the region [latex]D[/latex] in the [latex]xy[/latex]-plane which is the base of the parallelepiped: [latex]\iint_D 5 \ dx\, dy[/latex]
- By calculating the triple integral of the constant function 1 over the parallelepiped itself: [latex]\iiint_\mathrm{parallelepiped} 1 \, dx\, dy\, dz[/latex]
Example 2
Integrate [latex]f(x,y,z) = x^2 + y^2 + z^2[/latex] over the domain [latex]D = \left \{ x^2 + y^2 + z^2 \le 16 \right \}[/latex]. Looking at the domain, it seems convenient to adopt the passage in spherical coordinates; in fact, the intervals of the variables that delimit the new [latex]T[/latex] region are obviously: [latex-display](0 \le \rho \le 4, \ 0 \le \phi \le \pi, \ 0 \le \theta \le 2 \pi)[/latex-display] For the function, we get: [latex-display]f(\rho \sin \phi \cos \theta, \rho \sin \phi \sin \theta, \rho \cos \phi) = \rho^2[/latex-display] Therefore: [latex-display]\begin{align} \iiint_D (x^2 + y^2 +z^2) \, dx\, dy\, dz &= \iiint_T \rho^2 \ \rho^2 \sin \theta \, d\rho\, d\theta\, d\phi \\ &= \int_0^{\pi} \sin \phi \,d\phi \int_0^4 \rho^4 d \rho \int_0^{2 \pi} d\theta \\ &= 2 \pi \int_0^{\pi} \sin \phi \left[ \frac{\rho^5}{5} \right]_0^4 \, d \phi \\ &= 2 \pi \left[ \frac{\rho^5}{5} \right]_0^4 \left[- \cos \phi \right]_0^{\pi} = \frac{4096 \pi}{5} \end{align}[/latex-display]Change of Variables
One makes a change of variables to rewrite the integral in a more "comfortable" region, which can be described in simpler formulae.Learning Objectives
Use a change a variables to rewrite an integral in a more familiar regionKey Takeaways
Key Points
- There exist three main "kinds" of changes of variable (to polar coordinate in [latex]R^2[/latex], and to cylindrical and spherical coordinates in [latex]R^3[/latex]); however, more general substitutions can be made using the same principle.
- When changing integration variables, make sure that the integral domain also changes accordingly.
- Change of variable should be judiciously applied based on the built-in symmetry of the function to be integrated.
Key Terms
- polar coordinate: a two-dimensional coordinate system in which each point on a plane is determined by a distance from a fixed point and an angle from a fixed direction
1. Polar coordinates
The function to be integrated transforms as: [latex-display]f(x,y) \rightarrow f(\rho \cos \phi,\rho \sin \phi )[/latex-display] and the integral accordingly changes as: [latex]\displaystyle {\iint_D f(x,y) \ dx\, dy = \iint_T f(\rho \cos \phi, \rho \sin \phi) \rho \, d \rho\, d \phi}[/latex]2. Cylindrical coordinates
The function to be integrated transforms as: [latex-display]f(x,y,z) \rightarrow f(\rho \cos \phi, \rho \sin \phi, z)[/latex-display] and the integral accordingly changes as: [latex]\displaystyle {\iiint_D f(x,y,z) \, dx\, dy\, dz = \iiint_T f(\rho \cos \phi, \rho \sin \phi, z) \rho \, d\rho\, d\phi\, dz}[/latex]
Cylindrical Coordinates: Changing to cylindrical coordinates may be useful depending on the setup of problem.
3. Spherical coordinates
The function to be integrated transforms as: [latex-display]f(x,y,z) \longrightarrow f(\rho \cos \theta \sin \phi, \rho \sin \theta \sin \phi, \rho \cos \phi)[/latex-display] and the integral accordingly changes as: [latex]\displaystyle {\iiint_D f(x,y,z) \, dx\, dy\, dz \\ = \iiint_T f(\rho \sin \phi \cos \theta, \rho \sin \phi \sin \theta, \rho \cos \phi) \rho^2 \sin \phi \, d\rho\, d\theta\, d\phi}[/latex]Applications of Multiple Integrals
Multiple integrals are used in many applications in physics and engineering.Learning Objectives
Apply multiple integrals to real world examplesKey Takeaways
Key Points
- Given a set [latex]D \subseteq R^n[/latex] and an integrable function [latex]f[/latex] over [latex]D[/latex], the average value of [latex]f[/latex] over its domain is given by [latex]\bar{f} = \frac{1}{m(D)} \int_D f(x)dx[/latex], where [latex]m(D)[/latex] is the measure of [latex]D[/latex].
- The gravitational potential associated with a mass distribution given by a mass measure [latex]dm[/latex] on three-dimensional Euclidean space [latex]R^3[/latex] is [latex]V(\mathbf{x}) = -\int_{\mathbf{R}^3} \frac{G}{|\mathbf{x} - \mathbf{r}|}\,dm(\mathbf{r})[/latex].
- An electric field produced by a distribution of charges given by the volume charge density [latex]\rho (\vec r)[/latex] is obtained by a triple integral of a vector function: [latex]\vec E = \frac {1}{4 \pi \epsilon_0} \iiint \frac {\vec r - \vec r'}{\| \vec r - \vec r' \|^3} \rho (\vec r')\, {d}^3 r'[/latex].
Key Terms
- Maxwell's equations: a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits
- moment of inertia: a measure of a body's resistance to a change in its angular rotation velocity
Example 1
In mechanics, the moment of inertia is calculated as the volume integral (triple integral) of the density weighed with the square of the distance from the axis: [latex-display]\displaystyle{I_z = \iiint_V \rho r^2\, dV}[/latex-display]Example 2
The gravitational potential associated with a mass distribution given by a mass measure [latex]dm[/latex] on three-dimensional Euclidean space [latex]R^3[/latex] is: [latex-display]\displaystyle{V(\mathbf{x}) = -\int_{\mathbf{R}^3} \frac{G}{\left|\mathbf{x} - \mathbf{r}\right|}\,dm(\mathbf{r})}[/latex-display] If there is a continuous function [latex]\rho(x)[/latex] representing the density of the distribution at [latex]x[/latex], so that [latex]dm(x) = \rho (x)d^3x[/latex], where [latex]d^3x[/latex] is the Euclidean volume element, then the gravitational potential is: [latex-display]\displaystyle{V(\mathbf{x}) = -\int_{\mathbf{R}^3} \frac{G}{\left|\mathbf{x}-\mathbf{r}\right|}\,\rho(\mathbf{r})\,d^3\mathbf{r}}[/latex-display]
A Mass to be Integrated: Points [latex]\mathbf{x}[/latex] and [latex]\mathbf{r}[/latex], with [latex]\mathbf{r}[/latex] contained in the distributed mass (gray) and differential mass [latex]dm(\mathbf{r})[/latex] located at the point [latex]\mathbf{r}[/latex].
Example 3
In electromagnetism, Maxwell's equations can be written using multiple integrals to calculate the total magnetic and electric fields. In the following example, the electric field produced by a distribution of charges given by the volume charge density [latex]\rho (\vec r)[/latex] is obtained by a triple integral of a vector function: [latex-display]\displaystyle{\vec E = \frac {1}{4 \pi \epsilon_0} \iiint \frac {\vec r - \vec r'}{\| \vec r - \vec r' \|^3} \rho (\vec r')\, {d}^3 r'}[/latex-display] This can also be written as an integral with respect to a signed measure representing the charge distribution.Center of Mass and Inertia
The center of mass for a rigid body can be expressed as a triple integral.Learning Objectives
Use multiple integrals to find the center of mass of a distribution of massKey Takeaways
Key Points
- In the case of a single rigid body, the center of mass is fixed in relation to the body, and if the body has uniform density, it will be located at the centroid.
- In the case of a system of particles [latex]P_i, i = 1, \cdots, n[/latex], each with mass [latex]m_i[/latex] that are located in space with coordinates [latex]\mathbf{r}_i, i = 1, \cdots, n[/latex], the coordinates [latex]\mathbf{R}[/latex] of the center of mass is given as [latex]\mathbf{R} = \frac{1}{M} \sum_{i=1}^n m_i \mathbf{r}_i[/latex].
- If the mass distribution is continuous with the density [latex]\rho (r)[/latex] within a volume [latex]V[/latex], the center of mass is expressed as [latex]\mathbf R = \frac 1M \int_V\rho(\mathbf{r}) \mathbf{r} dV[/latex].
Key Terms
- rigid body: an idealized solid whose size and shape are fixed and remain unaltered when forces are applied; used in Newtonian mechanics to model real objects
- centroid: the point at the center of any shape, sometimes called the center of area or the center of volume

Center of Mass: Two bodies orbiting around the center of mass inside one body
A System of Particles
In the case of a system of particles [latex]P_i, i = 1, \cdots, n[/latex], each with mass [latex]m_i[/latex] that are located in space with coordinates [latex]\mathbf{r}_i, i = 1, \cdots, n[/latex], the coordinates [latex]\mathbf{R}[/latex] of the center of mass satisfy the condition: [latex-display]\displaystyle{\sum_{i=1}^n m_i(\mathbf{r}_i - \mathbf{R}) = 0}[/latex-display] Solve this equation for [latex]\mathbf{R}[/latex] to obtain the formula: [latex-display]\displaystyle{\mathbf{R} = \frac{1}{M} \sum_{i=1}^n m_i \mathbf{r}_i}[/latex-display] where [latex]M[/latex] is the sum of the masses of all of the particles.A Continuous Volume
If the mass distribution is continuous with the density [latex]\rho (r)[/latex] within a volume [latex]V[/latex], then the integral of the weighted position coordinates of the points in this volume relative to the center of mass [latex]\mathbf{R}[/latex] is zero; that is: [latex-display]\displaystyle{\int_V \rho(\mathbf{r})(\mathbf{r}-\mathbf{R})dV = 0}[/latex-display] Solve this equation for the coordinates [latex]\mathbf{R}[/latex] to obtain: [latex-display]\displaystyle{\mathbf R = \frac 1M \int_V\rho(\mathbf{r}) \mathbf{r} dV}[/latex-display] where [latex]M[/latex] is the total mass in the volume. The integral is over the three dimensional volume, so it is a triple integral.Licenses & Attributions
CC licensed content, Shared previously
- Curation and Revision. Provided by: Boundless.com License: CC BY-SA: Attribution-ShareAlike.
CC licensed content, Specific attribution
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Fubini's theorem. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- hypervolume. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Iterated integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Fubini's theorem. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- domain. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Polar coordinate system. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Jacobian determinant. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Cartesian. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- differential. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- cylindrical coordinate. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Jacobian determinant. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- spherical coordinate. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- spherical coordinate. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- polar coordinate. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Cylindrical Coordinates. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- Maxwell's equations. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- moment of inertia. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Cylindrical Coordinates. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Gravitational potential. Provided by: Wikipedia License: CC BY: Attribution.
- Center of mass. Provided by: Wikipedia License: CC BY-SA: Attribution-ShareAlike.
- centroid. Provided by: Wiktionary License: CC BY-SA: Attribution-ShareAlike.
- rigid body. Provided by: Wiktionary Located at: https://en.wiktionary.org/wiki/rigid_body. License: CC BY-SA: Attribution-ShareAlike.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Multiple integral. Provided by: Wikipedia License: CC BY: Attribution.
- Cylindrical Coordinates. Provided by: Wikipedia License: Public Domain: No Known Copyright.
- Gravitational potential. Provided by: Wikipedia License: CC BY: Attribution.
- Center of mass. Provided by: Wikipedia License: CC BY: Attribution.
