Polynomial Basics
Earl is building a doghouse, whose front is in the shape of a square topped with a triangle. There will be a rectangular door through which the dog can enter and exit the house. Earl wants to find the area of the front of the doghouse so that he can purchase the correct amount of paint. Using the measurements of the front of the house, shown in Figure 1, we can create an expression that combines several variable terms, allowing us to solve this problem and others like it.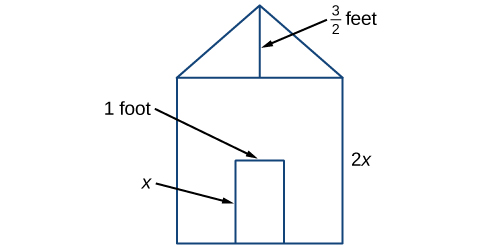 Figure 1
Figure 1Operations on Polynomials
The area of the front of the doghouse can be found by adding the areas of the square and the triangle, and then subtracting the area of the rectangle. When we do this, we get [latex]4{x}^{2}+\frac{3}{2}x-x{\text{ft}}^{2}[/latex], or [latex]4{x}^{2}+\frac{1}{2}x[/latex] ft2. In this section, we will examine expressions such as this one, which combine several variable terms. The area of the front of the doghouse described in the introduction was [latex]4{x}^{2}+\frac{1}{2}x[/latex] ft2. This is an example of a polynomial, which is a sum of or difference of terms, each consisting of a variable raised to a nonnegative integer power. A number multiplied by a variable raised to an exponent, such as [latex]384\pi [/latex], is known as a coefficient. Coefficients can be positive, negative, or zero, and can be whole numbers, decimals, or fractions. Each product [latex]{a}_{i}{x}^{i}[/latex], such as [latex]384\pi w[/latex], is a term of a polynomial. If a term does not contain a variable, it is called a constant. A polynomial containing only one term, such as [latex]5{x}^{4}[/latex], is called a monomial. A polynomial containing two terms, such as [latex]2x - 9[/latex], is called a binomial. A polynomial containing three terms, such as [latex]-3{x}^{2}+8x - 7[/latex], is called a trinomial. We can find the degree of a polynomial by identifying the highest power of the variable that occurs in the polynomial. The term with the highest degree is called the leading term because it is usually written first. The coefficient of the leading term is called the leading coefficient. When a polynomial is written so that the powers are descending, we say that it is in standard form.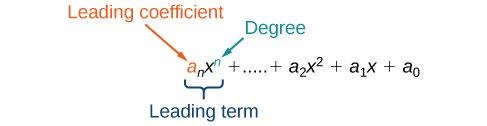
A General Note: Polynomials
A polynomial is an expression that can be written in the formHow To: Given a polynomial expression, identify the degree and leading coefficient.
- Find the highest power of x to determine the degree.
- Identify the term containing the highest power of x to find the leading term.
- Identify the coefficient of the leading term.
Example: Identifying the Degree and Leading Coefficient of a Polynomial
For the following polynomials, identify the degree, the leading term, and the leading coefficient.- [latex]3+2{x}^{2}-4{x}^{3}[/latex]
- [latex]5{t}^{5}-2{t}^{3}+7t[/latex]
- [latex]6p-{p}^{3}-2[/latex]
Answer:
- The highest power of x is 3, so the degree is 3. The leading term is the term containing that degree, [latex]-4{x}^{3}[/latex]. The leading coefficient is the coefficient of that term, [latex]-4[/latex].
- The highest power of t is [latex]5[/latex], so the degree is [latex]5[/latex]. The leading term is the term containing that degree, [latex]5{t}^{5}[/latex]. The leading coefficient is the coefficient of that term, [latex]5[/latex].
- The highest power of p is [latex]3[/latex], so the degree is [latex]3[/latex]. The leading term is the term containing that degree, [latex]-{p}^{3}[/latex], The leading coefficient is the coefficient of that term, [latex]-1[/latex].
Try It
Identify the degree, leading term, and leading coefficient of the polynomial [latex]4{x}^{2}-{x}^{6}+2x - 6[/latex].Answer: The degree is 6, the leading term is [latex]-{x}^{6}[/latex], and the leading coefficient is [latex]-1[/latex].
Example: Subtracting Polynomials
Find the difference.[latex]\left(7{x}^{4}-{x}^{2}+6x+1\right)-\left(5{x}^{3}-2{x}^{2}+3x+2\right)[/latex]
Answer: [latex-display]\begin{array}{cc}7{x}^{4}-5{x}^{3}+\left(-{x}^{2}+2{x}^{2}\right)+\left(6x - 3x\right)+\left(1 - 2\right)\text{ }\hfill & \text{Combine like terms}.\hfill \\ 7{x}^{4}-5{x}^{3}+{x}^{2}+3x - 1\hfill & \text{Simplify}.\hfill \end{array}[/latex-display]
Analysis of the Solution
Note that finding the difference between two polynomials is the same as adding the opposite of the second polynomial to the first.Try It
Find the difference. [latex-display]\left(-7{x}^{3}-7{x}^{2}+6x - 2\right)-\left(4{x}^{3}-6{x}^{2}-x+7\right)[/latex-display]Answer: [latex-display]-11{x}^{3}-{x}^{2}+7x - 9[/latex-display]
Using FOIL to Multiply Binomials
A shortcut called FOIL is sometimes used to find the product of two binomials. It is called FOIL because we multiply the first terms, the outer terms, the inner terms, and then the last terms of each binomial.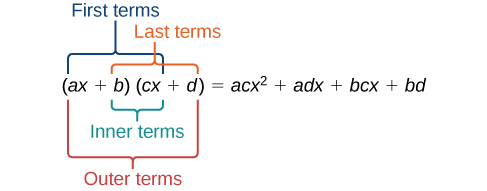 The FOIL method arises out of the distributive property. We are simply multiplying each term of the first binomial by each term of the second binomial, and then combining like terms.
The FOIL method arises out of the distributive property. We are simply multiplying each term of the first binomial by each term of the second binomial, and then combining like terms.
How To: Given two binomials, use FOIL to simplify the expression.
- Multiply the first terms of each binomial.
- Multiply the outer terms of the binomials.
- Multiply the inner terms of the binomials.
- Multiply the last terms of each binomial.
- Add the products.
- Combine like terms and simplify.
Example: Using FOIL to Multiply Binomials
Use FOIL to find the product.Answer:
Find the product of the first terms.
 Find the product of the outer terms.
Find the product of the outer terms.
 Find the product of the inner terms.
Find the product of the inner terms.
 Find the product of the last terms.
Find the product of the last terms.

[latex]\begin{array}{cc}6{x}^{2}+6x - 54x - 54\hfill & \text{Add the products}.\hfill \\ 6{x}^{2}+\left(6x - 54x\right)-54\hfill & \text{Combine like terms}.\hfill \\ 6{x}^{2}-48x - 54\hfill & \text{Simplify}.\hfill \end{array}[/latex]
Try It
Use FOIL to find the product. [latex-display]\left(x+7\right)\left(3x - 5\right)[/latex-display]Answer: [latex-display]3{x}^{2}+16x - 35[/latex-display]
Difference of Squares
Another special product is called the difference of squares, which occurs when we multiply a binomial by another binomial with the same terms but the opposite sign. Let’s see what happens when we multiply [latex]\left(x+1\right)\left(x - 1\right)[/latex] using the FOIL method.Q & A
Is there a special form for the sum of squares?
No. The difference of squares occurs because the opposite signs of the binomials cause the middle terms to disappear. There are no two binomials that multiply to equal a sum of squares.A General Note: Difference of Squares
When a binomial is multiplied by a binomial with the same terms separated by the opposite sign, the result is the square of the first term minus the square of the last term.How To: Given a binomial multiplied by a binomial with the same terms but the opposite sign, find the difference of squares.
- Square the first term of the binomials.
- Square the last term of the binomials.
- Subtract the square of the last term from the square of the first term.
Example: Multiplying Binomials Resulting in a Difference of Squares
Multiply [latex]\left(9x+4\right)\left(9x - 4\right)[/latex].Answer: Square the first term to get [latex]{\left(9x\right)}^{2}=81{x}^{2}[/latex]. Square the last term to get [latex]{4}^{2}=16[/latex]. Subtract the square of the last term from the square of the first term to find the product of [latex]81{x}^{2}-16[/latex].
Try It 7
Multiply [latex]\left(2x+7\right)\left(2x - 7\right)[/latex].Answer: [latex]4{x}^{2}-49[/latex]
Key Equations
| perfect square trinomial | [latex]{\left(x+a\right)}^{2}=\left(x+a\right)\left(x+a\right)={x}^{2}+2ax+{a}^{2}[/latex] |
| difference of squares | [latex]\left(a+b\right)\left(a-b\right)={a}^{2}-{b}^{2}[/latex] |
Key Concepts
- A polynomial is a sum of terms each consisting of a variable raised to a non-negative integer power. The degree is the highest power of the variable that occurs in the polynomial. The leading term is the term containing the highest degree, and the leading coefficient is the coefficient of that term.
- We can add and subtract polynomials by combining like terms.
- To multiply polynomials, use the distributive property to multiply each term in the first polynomial by each term in the second. Then add the products.
- FOIL (First, Outer, Inner, Last) is a shortcut that can be used to multiply binomials.
- Perfect square trinomials and difference of squares are special products.
- Follow the same rules to work with polynomials containing several variables.
Glossary
binomial a polynomial containing two terms coefficient any real number [latex]{a}_{i}[/latex] in a polynomial in the form [latex]{a}_{n}{x}^{n}+\dots+{a}_{2}{x}^{2}+{a}_{1}x+{a}_{0}[/latex] degree the highest power of the variable that occurs in a polynomial difference of squares the binomial that results when a binomial is multiplied by a binomial with the same terms, but the opposite sign leading coefficient the coefficient of the leading term leading term the term containing the highest degree monomial a polynomial containing one term perfect square trinomial the trinomial that results when a binomial is squared polynomial a sum of terms each consisting of a variable raised to a nonnegative integer power term of a polynomial any [latex]{a}_{i}{x}^{i}[/latex] of a polynomial in the form [latex]{a}_{n}{x}^{n}+\dots+{a}_{2}{x}^{2}+{a}_{1}x+{a}_{0}[/latex] trinomial a polynomial containing three termsLicenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
- Examples: Intro to Polynomials. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
- Examples: Adding and Subtracting Polynomials. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
- Examples: Multiplying Polynomials. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
- Question ID 93531, 93536, 93537, 93539. Authored by: Michael Jenck. License: CC BY: Attribution. License terms: IMathAS Community License, CC-BY + GPL.
- Question ID 3864. Authored by: Tyler Wallace. License: CC BY: Attribution. License terms: IMathAS Community License, CC-BY + GPL.
- Question ID 1825. Authored by: David Whittaker. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
- Question 1856. Authored by: Lawrence Morales. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
- Question ID 100774. Authored by: Rick Rieman. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
CC licensed content, Specific attribution
- College Algebra. Provided by: OpenStax Authored by: OpenStax College Algebra. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
